In probability theory, the craps principle is a theorem about eventprobabilities under repeated iid trials. Let ${\displaystyle E_1}$ and ${\displaystyle E_2}$ denote two mutually exclusive events which might occur on a given trial. Then the probability that ${\displaystyle E_1}$ occurs before ${\displaystyle E_2}$ equals the conditional probability that ${\displaystyle E_1}$ occurs given that ${\displaystyle E_1}$ or ${\displaystyle E_2}$ occur on the next trial, which is

• Conditional Probability Formula
• Craps Probabilities
• Craps Conditional Probability Test
• Conditional Probability Examples

However, what makes it cumbersome is a slightly complicated sample space and conditional probabilities! In this lesson, students learn about the game of craps as the avenue for some sophisticated theoretical probability calculations, and they learn why, in the long run, the house always wins. So to get a 6 when rolling a six-sided die, probability = 1 ÷ 6 = 0.167, or 16.7 percent chance. Independent probabilities are calculated using: Probability of both = Probability of outcome one × Probability of outcome two. So to get two 6s when rolling two dice, probability = 1/6 × 1/6 = 1/36 = 1 ÷ 36 = 0.0278, or 2.78 percent.

The craps dice odds and probabilities list as follows: 2 and 12 have only one way they can be formed on two dice, thus carrying odds of 35 to 1 (a one in thirty-six chance of being rolled). 3 and 11 have two possible formations, so the odds of these appearing are 17 to 1. The probability of winning on a 4 or 10 in craps is (6/36)×(3/9) = 5.56%. Every time this happens you get an extra unit, so it is worth 5.56%. Normally the house edge on the come bet is 1.41%, so overall the player edge under this rule is 4.15%.

${\displaystyle \mathrm{P}[E_1\text{before}{E}_2]=\mathrm{P}\left[E_1\mid E_1\cup E_2\right]=\frac{\mathrm{P}[E_1]}{\mathrm{P}[E_1]+\mathrm{P}[E_2]}}$

The events ${\displaystyle E_1}$ and ${\displaystyle E_2}$ need not be collectively exhaustive (if they are, the result is trivial).^[1][2]

Proof[edit]

Let ${\displaystyle A}$ be the event that ${\displaystyle E_1}$ occurs before ${\displaystyle E_2}$. Let ${\displaystyle B}$ be the event that neither ${\displaystyle E_1}$ nor ${\displaystyle E_2}$ occurs on a given trial. Since ${\displaystyle B}$, ${\displaystyle E_1}$ and ${\displaystyle E_2}$ are mutually exclusive and collectively exhaustive for the first trial, we have

${\displaystyle \mathrm{P}(A)=\mathrm{P}(E_1)\mathrm{P}(A\mid E_1)+\mathrm{P}(E_2)\mathrm{P}(A\mid E_2)+\mathrm{P}(B)\mathrm{P}(A\mid B)=\mathrm{P}(E_1)+\mathrm{P}(B)\mathrm{P}(A\mid B)}$

and ${\displaystyle \mathrm{P}(B)=1-\mathrm{P}(E_1)-\mathrm{P}(E_2)}$. Since the trials are i.i.d., we have ${\displaystyle \mathrm{P}(A\mid B)=\mathrm{P}(A)}$. Using ${\displaystyle \mathrm{P}(A{E}_1)=1,\mathrm{P}(A{E}_2)=0}$ and solving the displayed equation for ${\displaystyle \mathrm{P}(A)}$ gives the formula

${\displaystyle \mathrm{P}(A)=\frac{\mathrm{P}(E_1)}{\mathrm{P}(E_1)+\mathrm{P}(E_2)}}$.

Application[edit]

If the trials are repetitions of a game between two players, and the events are

${\displaystyle E_1:\mathrm{p}\mathrm{l}\mathrm{a}\mathrm{y}\mathrm{e}\mathrm{r}1\mathrm{w}\mathrm{i}\mathrm{n}\mathrm{s}}$

${\displaystyle E_2:\mathrm{p}\mathrm{l}\mathrm{a}\mathrm{y}\mathrm{e}\mathrm{r}2\mathrm{w}\mathrm{i}\mathrm{n}\mathrm{s}}$

then the craps principle gives the respective conditional probabilities of each player winning a certain repetition, given that someone wins (i.e., given that a draw does not occur). In fact, the result is only affected by the relative marginal probabilities of winning ${\displaystyle \mathrm{P}[E_1]}$ and ${\displaystyle \mathrm{P}[E_2]}$ ; in particular, the probability of a draw is irrelevant.

Stopping[edit]

If the game is played repeatedly until someone wins, then the conditional probability above is the probability that the player wins the game. This is illustrated below for the original game of craps, using an alternative proof.

Craps example[edit]

If the game being played is craps, then this principle can greatly simplify the computation of the probability of winning in a certain scenario. Specifically, if the first roll is a 4, 5, 6, 8, 9, or 10, then the dice are repeatedly re-rolled until one of two events occurs:

${\displaystyle E_1:\text{the original roll (called ‘the point’) is rolled (a win)}}$

${\displaystyle E_2:\text{a 7 is rolled (a loss)}}$

Since ${\displaystyle E_1}$ and ${\displaystyle E_2}$ are mutually exclusive, the craps principle applies. For example, if the original roll was a 4, then the probability of winning is

${\displaystyle \frac{3/36}{3/36+6/36}=\frac{1}{3}}$

This avoids having to sum the infinite series corresponding to all the possible outcomes:

${\displaystyle \sum _{i=0}^{\mathrm{\infty }}\mathrm{P}[\text{first i rolls are ties,}(i+1{)}^{\text{th}}\text{roll is ‘the point’}]}$

Mathematically, we can express the probability of rolling ${\displaystyle i}$ ties followed by rolling the point:

${\displaystyle \mathrm{P}[\text{first i rolls are ties,}(i+1{)}^{\text{th}}\text{roll is ‘the point’}]=(1-\mathrm{P}[E_1]-\mathrm{P}[E_2]{)}^i\mathrm{P}[E_1]}$

Conditional Probability Formula

The summation becomes an infinite geometric series:

${\displaystyle \sum _{i=0}^{\mathrm{\infty }}(1-\mathrm{P}[E_1]-\mathrm{P}[E_2]{)}^i\mathrm{P}[E_1]=\mathrm{P}[E_1]\sum _{i=0}^{\mathrm{\infty }}(1-\mathrm{P}[E_1]-\mathrm{P}[E_2]{)}^i}$

${\displaystyle =\frac{\mathrm{P}[E_1]}{1-(1-\mathrm{P}[E_1]-\mathrm{P}[E_2])}=\frac{\mathrm{P}[E_1]}{\mathrm{P}[E_1]+\mathrm{P}[E_2]}}$

which agrees with the earlier result.

References[edit]

1. ^Susan Holmes (1998-12-07). 'The Craps principle 10/16'. statweb.stanford.edu. Retrieved 2016-03-17.
2. ^Jennifer Ouellette (31 August 2010). The Calculus Diaries: How Math Can Help You Lose Weight, Win in Vegas, and Survive a Zombie Apocalypse. Penguin Publishing Group. pp. 50–. ISBN978-1-101-45903-4.

Craps Probabilities

Notes[edit]

• Pitman, Jim (1993). Probability. Berlin: Springer-Verlag. p. 210. ISBN0-387-97974-3.

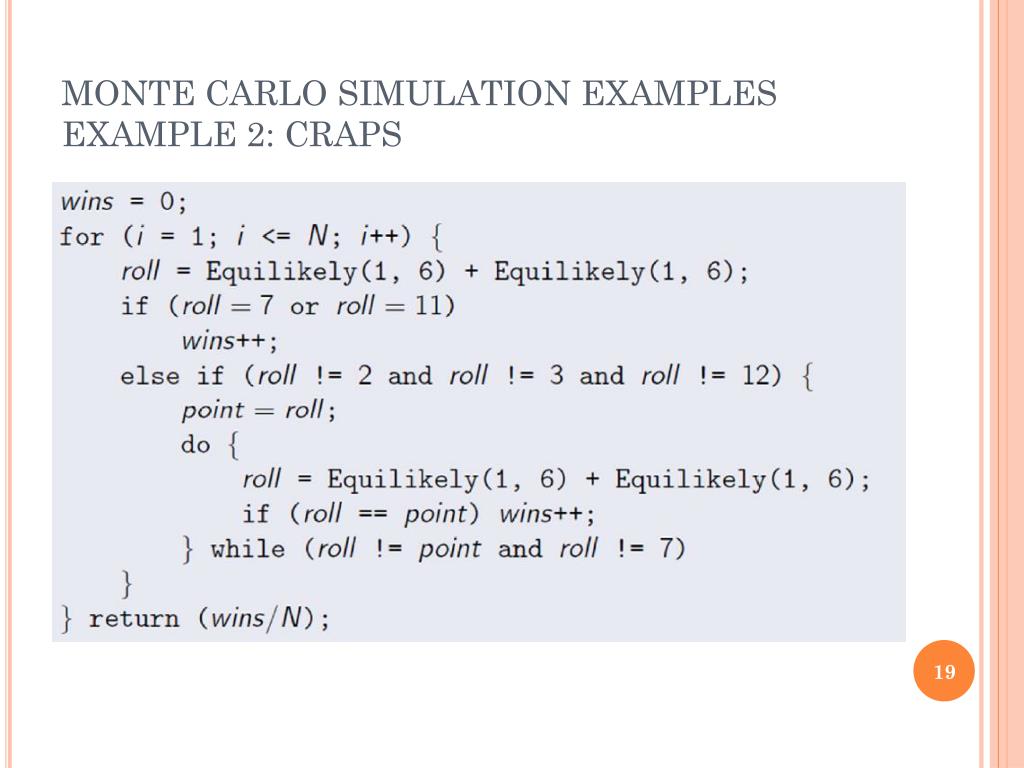
A popular dice game, called 'craps,' is played in the following manner. A player starts by rolling two dice. If the result is a 7 or 11, the player wins. If the result is 2, 3, or 12, the player loses. For any other sum appearing on the dice, the player continues to roll the dice until that outcome reoccurs (in which case the player wins) or until a 7 occurs (in which case the player loses).

a) What is the probability that a player wins the game on the first roll of the dice?
b) What is the probability that a player loses the game on the first roll of the dice?
c) If the player throws a 4 on the first roll, what is the probability that the game ends on the next roll?
d) If the player throws a 4 on the first roll, what is the probability that he will eventually win the game?

Craps Conditional Probability Test

Solution Preview

Conditional Probability Examples

Please see the attached Word document to view the table described in this solution.

The values 1 through 6 on the left and top margins of the table are the possible outcomes of one die. The body of the table contains the sum of the two values in the margins, the total when two dice are rolled.

There are 36 equally likely outcomes when two dice are rolled. The probability of an event when rolling a pair of dice can be determined by counting the ...

The solution gives an intuitive approach to probability problems involving dice. The technique is illustrated by calculating probabilities for the game of 'craps'.